Interpreting Shared DNA as Relationship

One result of a genetic genealogy test is the amount of DNA shared with another tester expressed in either centimorgans (cM) or percent.

How does that amount of shared DNA translate into the relationship? Sadly it's not unique and the smaller the amount shared the broader the range of possible relationships it implies. The relationship can only be expressed as a probability.

Suppose based on the amount of shared DNA, say 200 cM, there's a probability the relationship could be second cousin. It's a conditional probability which statisticians write as P(A|B) where A is the second cousin relationship and B the 200 cM of shared DNA.

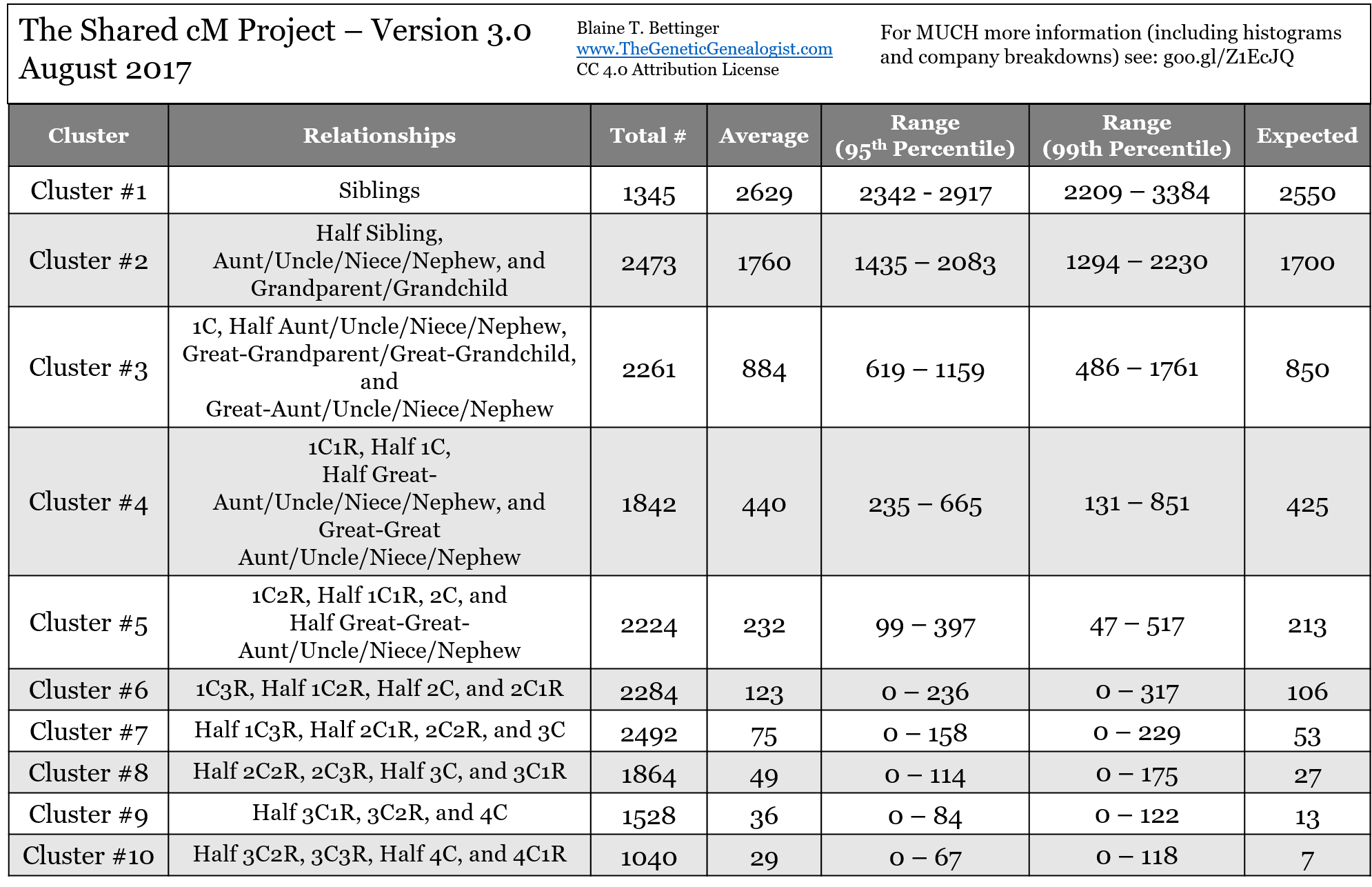
To help the interpretation Blaine Bettinger's Shared Centimorgan Project compiled statistics of the distribution of shared cM for a given relationship. Using the same terminology, it's expressed as the conditional probability P(B|A).

P(B|A) is not necessarily the same thing as P(A|B). If it were the probability that a black animal is a dog would equal the probability a dog is a black animal.

Bayes Theorem tells us how P(A|B) and P(B|A) are related. P(A) and P(B) are the probabilities of observing A (the relationship) and B (the amount of shared DNA) independently of each other; known as marginal probabilities. Only if P(A) = P(B) will P(A|B) = P(B|A).

How do you determine the marginal probabilities? You establish an unbiased domain which is the same for both P(A) and P(B). A community known to dogs that are black would not be unbiased.

The domain could be the world. How many second cousins do you have? Perhaps 100? With a world population of 7.7 billion the marginal probability P(A) = 100/7.7 billion = 1.3 x 10E-7. But with how many people in the world do you share 200 cM? As only a few tens of millions of people have taken a DNA test, and a large fraction of those are in the USA  how do you know the worldwide figure? You don't.

You might choose a domain such as all people who have taken a DNA test or tested with a particular company. In theory, you might know how many of those match at 200 cM (within a narrow range) but how would you know how many second cousins tested?

You might choose the domain of all those who responded to the Shared cM Project call. Everyone who did so was able to confirm the shared cM for a particular relationship. For a second cousin, the average was 233 cM with a range of 46 to 515 cM. The project found 18 other relationships where 200 cM was within the range of shared cM values found.

However, there's evidence that the Shared cM Project dataset is not an unbiased domain. The average amount of DNA for a given relationship is higher than would be expected from theory. That's shown by comparing the fourth and the final column in the table. And in terms of percent, the difference becomes more significant the more distant the relationship. That's what would be expected if one failed to establish a link when it existed. The capability, and maybe motivation to establish it is less for less shared DNA, especially for cases where the relationship is more distant and possibly obscured behind a genealogical brick wall or hidden by misattributed parentage.

Another factor is endogamy. Specific results in Table 3 at https://thegeneticgenealogist.com/wp-content/uploads/2017/08/Shared_cM_Project_2017.pdf show that people from an endogamous population share more DNA than would be expected from the closest relationship as they are also more distantly related. The Shared cM survey shows that for a fourth cousin, where the expected shared DNA is 13 cM, those from non-endogamous populations share an average of 33 cM while endogamous populations share an average of 53 cM.

While looking further into this I noticed the ratio of the number of endogamous to non-endogamous cases reported changes systematically as the relationship becomes more distant. For 1st cousins, endogamous cases are 15% of the total, for 2nd cousins 12%, 3rd cousins 10% and 4th cousins 9%. Why would endogamy decrease for more distant relationships? Likely people don't know about endogamy for more distant relatives.

If you read this far — congratulations. The bottom line is the marginal probabilities P(A) and P(B) are not equal, although for larger amounts of shared DNA they may be close enough that the difference is insignificant. Moving to smaller amounts of shared DNA relationships are likely more distant than the assumption P(A|B) = P(B|A) often applied to the Shared cM Project sample implies.